Buoyant Economies
Money and Inflation
This page attempts to explain and derive mathematically the relationship found to exist in the economy and presented in equation (12) and the chart below. Simply put, this approach assumes that money is necessary to repay debts and the value of the products supplied to earn the money necessary to repay debts determines the value of money, or prices.
The money that people and businesses hold represents their entitlements. What they can buy with that money is determined by prices. This can be written as follows:
At = Lt/Pt (1)
Where At is the value of entitlements in the economy at time "t";
Lt is the money supply (the total amount of money in the economy) at time "t"; and
Pt are average prices at time "t".
Let us assume that the bank loans that must be repaid at time "t" is a proportion of the money supply, which can be put as:
Bt = aLt (2)
Where Bt is the total amount of money that must be repaid it time "t"; and
"a" is the proportion of the money supply that must be repaid. For the purpose of this explanation, we will assume that there is no interest and the repayment is the repayment of the principal of the loan.
To repay the loan, the borrower must supply products to the economy with which to earn money to repay the loan. The amount of goods that they would have to supply depends upon the price. We can rewrite equation (2) in terms of real products and prices as follows:
Bt = bYtPt (3)
Where Yt is the quantity of goods and services produced in time "t"; and
"b" is the share of production that is to be sold to repay loans in time "t".
From equation (3) we can write that the total value of production supplied to the economy is given by:
Bt/b = YtPt (4)
What is being implied here, is that the prices that debtors supply products to the market to repay their debts, determines the level of prices in the economy. However, this is the same as saying that the prices in the economy determine the quantity of products that debtors have to supply (or sell) to the market to earn the money to repay their debt.
For the economy to be balanced (in equilibrium) what people can demand or buy with their money (i.e., total entitlements) given in equation (1), must equal what is available to be purchased or the value of production given in equation (4). That is:
Bt/b = At (5)
Therefore, substitution equations (1) and (4) into equation (5) tells us that production (or supply) at current prices must equal entitlements or demand at current prices:
YtPt = Lt/Pt (6)
Rearranging equation (6) it is possible to determine the level of prices to clear the market:
Pt2 = Lt/Yt
Pt = Ö(Lt/Yt) (7)(If you see an "H" in this equation it should be a square root sign.)
To make the formula for prices in equation (7) explain the change in prices over time or inflation, we will assume from equation (1) the following:
A0 = L0/P0 (8)
From equation (1) and (8) we define the change in the value of entitlements, or demand in the economy, as:
At/A0 = (Lt/L0)/(Pt/P0) (9)
Similarly, from equation (4) assuming that the share of production that is used to repay loans stays the same, we can do the same as in equation (9) and say that the growth in the value of goods produced in the economy (the supply side) is equal to the growth in the amount produced times the increase in prices, or inflation. That is:
(Bt/b)/ (Bo/b) = (Yt/Y0)(Pt/P0) (10)
As in equation (5), for market to be cleared , or at equilibrium, the growth in value of total production supplied from time "0" to time "t" as shown in equation (10) must equal the growth in the value of total entitlements over the same time period. That is:
(Bt/b)/(Bo/b) = At/A0 (11)
Substituting equations (9) and (10) in equation (11) provides the equation for inflation.
(Yt/Y0)(Pt/P0) = (Lt/L0)/(Pt/P0)
(Pt/P0)2 = (Lt/L0)/(Yt/Y0)
(Pt/P0) = Ö((Lt/L0)/(Yt/Y0)) (12) (If you see an "H" in this equation it should be a square root sign.)
This relationship can be confirmed with the data. Graphs of this relationship are available for Australia, the USA and New Zealand. Links are also available to the Microsoft Excel files with the data and graphs used to derive the graphs for Australia, the USA and New Zealand. Below is a chart of the official CPI for the USA and the CPI for the USA modelled using equation (12).
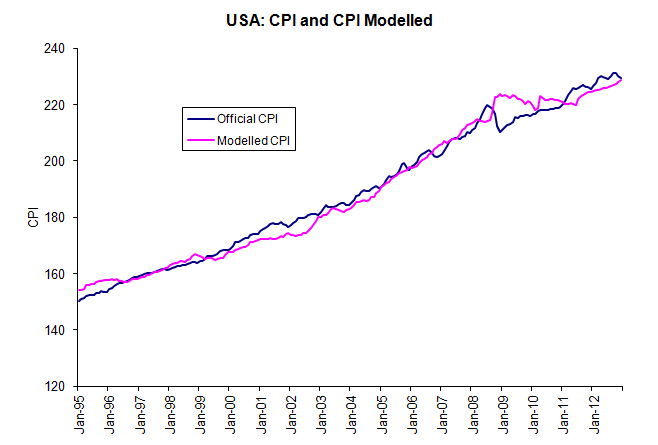
To see more about the implications of equation (12) for the sustainability of debt levels, see Money and Unsustainable Debt.
Note that before 1995, in the USA, the monetary policy was different and this equation does not hold. See the page on Money and Unsustainable Debt.
Reconciling with quantity theory
The quantity theory of money has indicated that there should be a proportional relationship between money and prices. This theory has said that
MV = PT (13)
Where M is the money supply;
V is the velocity of circulation;
P is prices; and
T is an index of the real value of transactions.
It has generally been assumed that "V", the velocity of circulation, is relatively constant and "T" is indicative of the level of real income. However, to reconcile the theory explained by equation (12) with that of the quantity theory, it is necessary to consider that money and transactions are necessary to repay debt.
For example, assume that a country starts with income of $1 B and money supply of $1 B. If real national income doubles, then the money supply would need to double, also, to $2 B. If prices double, also, then the country needs another $2 billion of money. If all money is created by the banks, then the the country has $4 B of debt. In addition, it will have foreign debt of $4 billion. It appears that to service these debts, the country needs that much money again, taking the total money supply to $8 billion.
This is the outcome as presented in equation (12). Hence it is possible to envisage a situation where the quantity theory and the theory represented by equation (12) are consistent.
To read more about similar issues, look up the papers on the subject.
Last update: 9 February 2013