Introduction

This paper presents models that explain how growth in the quantity of money determines the current account balance.  Money should constrain an economy's expenditure to its income.  If the only source of money were from income, then a country could not buy more than it produced; it would generally have a surplus or balanced current account.  This paper explains how current account deficits are caused when additional money is created which finances national expenditure in excess of national income (production). 

Considered are three sources of additional money: 

• additional money that arises from national savings when foreign income (e.g., from exports) is greater than expenditure on foreign goods and services (e.g., on imports);

• additional money from the growth of bank lending; and

• additional money from net foreign capital inflow.

 Also. considered are three means of reducing the quantity of money:

• reduced money when expenditure on foreign goods and services is greater than foreign income;

• reduced money when loans are repaid to banks; and 

• reduced money when there is a net international capital outflow.

It models the effect of these sources of money for countries with fixed exchange rates (Figures 1-7) and those with floating exchange rates (Figures 7-13). Finally, there is a model of an integrated monetary and exchange rate system that manages the current account balance (Figures 14 and 15).

Background to the formula

The following analysis is based upon the experience of Leigh Harkness when he was the economist in the Ministry of Finance for the small South Pacific Island country of Tonga.   

Up until 1974, the Kingdom of Tonga did not have a bank.  Even so, the country issued its own currency.   When foreign currency entered the country, the government would exchange that money for domestic currency and add the foreign money to the country’s foreign reserves.  When people spent money on imports, the government converted their domestic currency back to foreign currency to pay for the imports.  In that way the country always had sufficient foreign reserves to pay for its imports.

When Tonga established a bank, it started lending money.  That lending created additional money that did not contribute to foreign reserves.  Yet, when that money was spent on imports, it still needed to be converted into foreign currency.  Therefore, increased bank lending depleted the country’s foreign reserves. 

In December 1981, to ensure that there were adequate foreign reserves to meet requirements, the government advised the bank to regulate its lending according to the level of foreign reserves.  If there were plenty of foreign reserves, it could lend without restraint.  But if foreign reserves were low, it was required to slow down or stop lending.  The International Monetary Fund later commended the government on the success of that policy. 

Leigh Harkness joined the Australian Treasury in July 1984.  Australia had large current account deficits.  He looked into whether bank credit could explain the current account deficit in Australia, as it had in Tonga.  He found that the relationship between bank credit and the current account deficit was even stronger in Australia than the relationship between bank credit and foreign reserves in Tonga. 

An Analogy - Cause and effect

To understand what causes current account deficits, consider the following simple analogy.  If an airline has an aircraft with 100 seats and sells 150 tickets for a flight, how many seats will it be short by? Obviously 50.  It is the same with the economy.   Current account deficits occur when there are more tickets (money) issued than there are goods. 

To solve the seating problem, should the airline buy a bigger plane?  If it buys a 150 seat aircraft and now sells 250 seats for a flight, has the problem been solved?  Of course not.  The deficiency has increased to 100 seats.  

The solution for the airline is in its ticketing system: it must link the availability of tickets to the availability of seats.  Likewise, the solution for an economy is to link the creation of additional money (which represents current entitlements to goods and services) to the availability of additional current obligations to supply goods and services.

The void of economic theory

There is not a widely accepted theory for the cause of a current account deficit. Many economists believe that people and businesses choose to have current account deficit.  That is, they want to borrow more money now and so are prepared to build up foreign debt which they will repay in the future. 

Also, many economists believe that financial institutions, pursuing their own interests, will achieve optimal outcomes for the economy.  While the Global Financial Crisis may have revealed that some regulation may be necessary to avoid abuse by delinquent financial institutions, most of these economists continue to cling to their faith in the current financial system. 

Keynesian economic theory had defined the current account balance as the difference between saving and investment.  However, from the perspective of the national economy, the reduction in spending of savers within the economy is offset by the equivalent increase in spending by borrowers.  These transfers within the economy do not affect the current account balance.

From the perspective of the current account balance, it is spending that exceeds income that is relevant.  It is irrelevant to the current account balance whether that excess spending was directed at consumption or investment.

Keynes had defined bank lending (a process that injects new funds into the economy) as "investment".  Also, he called the act of creating real capital as "investment".  At the time Keynes wrote, bank lending was used mainly for real capital investment.  Therefore, it may have been reasonable to use the same word for the two concepts.  However, in more recent times, the bank credit has been used for much more than new capital investment.  As a result, the meaning of the word "investment" has become ambiguous.  It can mean an increase in bank credit and debt, or it can mean an increase in real capital. Consequently, in the analysis below I have limited the use of the word "investment" to mean "bank credit" or "bank lending".

Currency and the current account deficit

This paper explains that the current account balance depends upon the rules that govern the monetary system, particularly the growth of bank credit. People have the option of borrowing and repaying their own debts.  But in certain cases, the country as a whole has no option but to go deeper and deeper into debt.  It is a consequence of their monetary system. 

In the monetary system, currency is a record or account of current entitlements.  Counterbalancing that record should be records or accounts of current obligations.   

Bonds are records of future entitlements.  Counterbalancing those future entitlements are obligations to pay in the future.  Those obligations are tied to the people that issued the bonds.  Without that obligation, the bonds would be worthless.

The obligation for currency is not tied to a particular person; it is linked to the obligations of the whole monetary system.  If currency is created without a corresponding current obligation, it affects all currency and the whole monetary system.   

Currency is used as a medium of exchange to buy and sell products.  Bonds may be exchanged for currency and, occasionally, they may be accepted in exchange for products.  But whoever accepts bonds in exchange for products recognizes that they cannot be used to pay wages and suppliers. The bonds must be sold in exchange for currency before the proceeds of the products sold are available to be spent.  Bonds are not money.

If currency is issued against future obligations, it creates additional current entitlements but there are not any additional current obligations to supply the products demanded.  The additional money will cause the economy to buy more than it has produced, unless there are offsetting national savings. That is, without offsetting savings, it will cause current account deficits.

Bank lending creates current entitlements against future obligations. The deregulation of the banking system has enabled banks to increase their lending.  That has increased the amount of current entitlements issued against future obligations.  It is this growth in bank credit has led to a rise in current account deficits.

Banks' books may appear balanced with debits equal to credits.  However, in terms of timing, the entitlements that they grant and the corresponding obligations are not synchronized.  Imports are required to satisfy the additional demand for the time between when the current entitlements were issued and when the loan was repaid.

As total bank lending has been rising, it means that new loans have been issued faster than old debt has been repaid.  Therefore, current account deficits have persisted and been equal to the growth in total bank credit, less any national savings.

National savings are not superannuation or deposits in bank accounts.  They are the nation’s income from the sale of exports that has not been spent on imports.  Essentially, they comprise foreign capital investments (in other economies) and the foreign reserves of the banking system.

In the days of fixed exchange rates, nations built up foreign reserves and saved.  But deregulation of the banking system caused those savings to decline.  The currency had to be floated to protect those reserves.  When a currency is floated, the whole financial system is required to balance international receipts and payments.  The monetary system no longer draws upon, nor contributes, to national savings.

Therefore, unless private international savings are greater than the growth of bank credit (as in Japan), countries with floating exchange rates will experience current account deficits equal to the growth of bank credit. 

The reality of that relationship is clearly evident in the following chart of the growth of bank credit and the current account deficit in the United States of America.  The amount of bank credit has been calculated from official US statistics.  A spreadsheet with the data is available here.

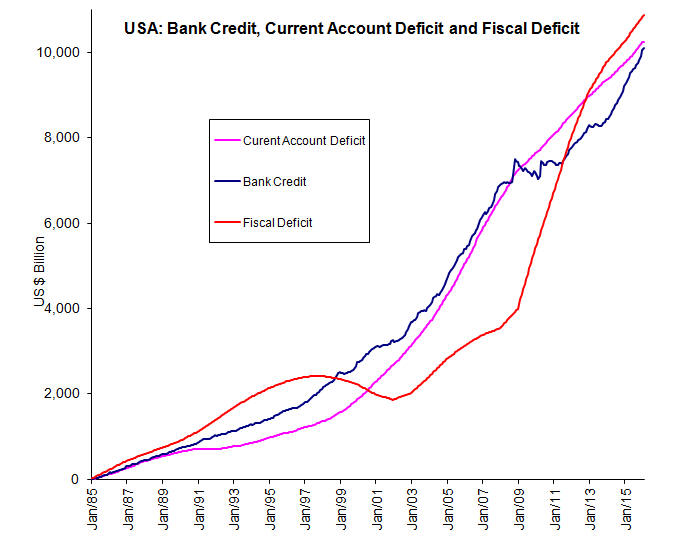
A similar chart is available below for New Zealand.  

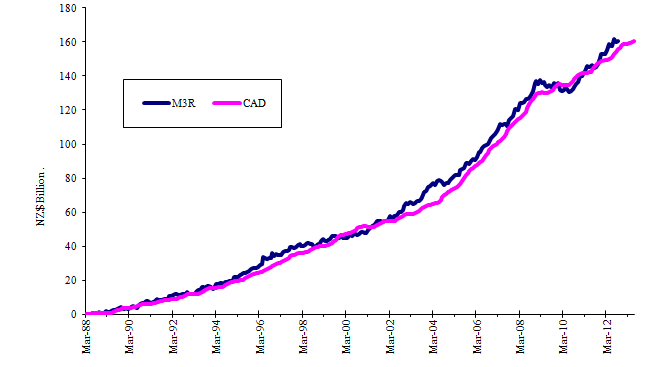
This graph clearly shows that the amount by which New Zealanders have bought more than they have produced is equal to the amount of additional money created from domestic sources.  The Reserve Bank of New Zealand identifies this excessive amount of additional money in its monetary statistics and calls it M3R money. The data for this graph is available here. 

The same relationship between the current account deficit and domestic sources of money can be found in other countries such as Australia, India and the Philippines.  As for the USA, the central banks of these countries have not specifically identified money from domestic sources.  Even so, that money can be extrapolated from the available statistics. 

When we spend the money that we have earned, it does not cause us to buy more than we have produced. Such spending does not lead to current account deficits.  The money we earn is like a receipt from the economy for supplying products and that money entitles us to buy products from the economy up to the value of what we have produced.  This system ensures that those with money have something to buy.  To use our airline analogy, it ensures that those who have tickets have a seat. 

To start identifying a formula for the current account deficit, we will start with sources of money that do not cause current account deficits.  But first, we need to clarify what we mean by the quantity of money.  

The Quantity of Money

Economists tend to talk of the quantity of money in terms of "the supply of" and "the demand for" money.  However, the amount of money in the economy is relatively simple to explain.  Regardless of whether it is supplied or demanded, there is a certain quantity of money in the economy and that is the money that we will deal with.  It consists of bank deposits (excluding saving bank deposits), other negotiable bank instruments (including bills of exchange) and currency (notes and coins). 

Money from foreign reserves

The spreadsheets with the models used to generate the following graphs are available in the "CAD Formula" Excel Workbook.  If there is any confusion as to what is meant by the following equations, look at the formulas in the spreadsheet model.  The models are intended to explain the relevant economic and financial principles.  They are not intended to represent any particular economy. 

To start building a formula for the current account deficit, let us assume that an economy has a fixed exchange rate and it's only source of money is from the growth of foreign reserves.  These foreign reserves accrue when the money earned from exports and other foreign sources is greater than the money spent on imports and other payments to foreign entities.  That is:

 R_t = R_t-1  + X_t-1  - M_t-1                                                  (1)                          

Where:

R_t is the level of foreign reserves at time “t”;

R_t-1 is the previous level of foreign reserves or the foreign reserves at time “t-1”;

X_t-1  are exports and other foreign receipts in time “t-1”; and

M_t-1  are imports and other foreign payments in time “t-1”

The foreign reserves are assets of the banking system.  Money is on the liabilities side of the banks’ balance sheets.  When banks buy foreign currency from exporters, they give them domestic currency in exchange.  Both currency and bank deposits are liabilities of the banking system.

As far as people in the economy are concerned, they earn money either from selling products to the domestic market or selling products overseas as exports.  Therefore, the quantity of money in the economy that they have to spend can be given by:  

L_t = N_t-1  + X_t-1                                                            (2)      

Where:

L_t is the quantity of money in the economy at time “t” available to be spent;

N_t-1 income from the sale of products to the domestic economy in time “t-1”.

To simplify the model, we will assume that “t” is the time taken to earn the amount of money L_t.  In that way, we can treat L_t not only as a stock of money but as the flow of money earned from domestic sales and from exports.  Therefore, we can say that:

Y_t = N_t  + X_t                                                                (3)      

Where:

Y_t is the income earned in time “t”.

We will assume that people spend their income or money on either domestic products or imports.  That is:

L_t = N_t  + M_t                                                                 (4)      

The quantity of money and income will continue to grow while exports are greater than imports. 

Let us assume that spending on imports is a proportion of total spending such that:

M_t = mL_t                                                                      (5)      

Where:

“m” is the proportion of total available money spent on imports or the marginal propensity to import.

By substituting equation (2) [L_t = N_t-1 + X_t-1] into equation (5) and the result into equation (4), we can conclude that:

L_t = N_{t  +} m(N_t-1   + X_t-1)                                               (6)      

Figure 1 below diagrammatically represents this formula. It assumes that exports are $5 B and the value of “m”, the marginal propensity to import, is 0.25 or 25%.  In the first period, there is no money to spend so nothing is spent on imports or domestic products.  In the second period, there is only the $5 B earned from exports to spend.  Of this, $1.25 B is spent on imports and $3.75 B on domestic products.  In the third period, there is $8.75 B (5+3.75) to spend.        

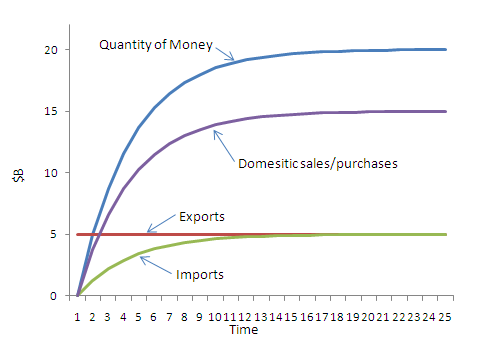
Figure 1: Foreign source of money

The quantity of money continues to grow, but more slowly, until it approaches $20 B.  When the quantity of money reaches $20 B, imports would be $5 B and equal to exports.  At this point, the quantity of money cannot increase any further.  It is said to be at equilibrium.  This equilibrium quantity of money can be defined as:

        L^* = X/m                                                                       (7)

Where L^* is the equilibrium quantity of money.

The quantity of money at a time “t” is defined as the income from domestic sales and foreign sources.  If the quantity of money is in equilibrium, then income is also at equilibrium.  In other words, the economy grows while it is in disequilibrium.  When the economy is at equilibrium, it stops growing.

Note that the current account has been in surplus or balanced throughout this example.  If the only source of money is from the growth of foreign reserves generated by exports, the current account will be balanced or in surplus. 

In this example, all additional money is created through national savings.  Also, the growth in the quantity of money in any period is equal to the current account balance.  

It was not necessary for any individual to defer spending for the nation to accumulate national savings. National savings occurred because the nation deferred spending on imports and spent that money on domestic products instead.    

Money from bank credit

Money can also be created through the growth of bank credit. The growth in bank credit is equal to new loans less loan repayments.  If we assume that bank credit is the only source of new money and there is no international trade, then we can say that the quantity of money in the economy can be given by:  

L_t = N_t-1  + C_rt   -  A_t                                                    (8)      

Where:

C_rt is the amount of new bank lending in time “t”;

A_t is the total amount of loan repayments in time “t”.

As all money here is created by bank credit, all money is equivalent to the outstanding loans.  We will assume that loan repayments in each period are a proportion of outstanding debt, such that:

Where:

a  is the proportion of outstanding debt repaid in a period.  It is the inverse of the average term of outstanding loans.

Substituting equation (9) into equation (8) we can conclude that:

This formula defines the quantity of money in time "t" as equal to the money earned from selling products in the domestic economy, plus new bank loans less loan repayments.  This domestic source of money is represented in Figure 2.  In this example, loans are assumed to be $5 B per period (C_rt = $5 B) and loan repayments in each period are 25% of the outstanding balance at the end of the previous period (a= 0.25).

 

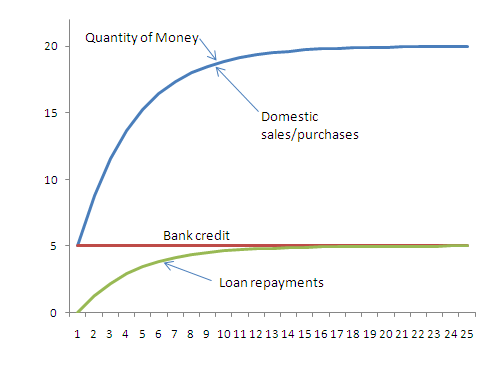
Figure 2: Domestic source of money

In this example, the equilibrium quantity of money is $20 B.  That is, total lending will be stable when loan repayments equal new lending.  That occurs when the quantity of bank debt, or money, is $20 B.  The equilibrium quantity of money from domestic sources (bank credit) is given by the following equation:

This model is a purely theoretical one of an economy with money.  It does not consider the effect of international trade.  Hence, there is no current account balance.  Even so, the equilibrium quantity of money from domestic sources is useful concept in explaining the cause of the current account balance.  

Money from foreign and domestic sources

In a country with both foreign and domestic sources of money the total quantity of money is the sum of both sources.  That is:

L_t = L_ft + L_ct                                                                     (12)

Where:

L_ft is the quantity of money from foreign sources and from equation (2) [L_t = N_t-1  + X_t-1] may be written as:

L_ft = N_t-1  + X_t-1                                                              (13)    

L_ct is the quantity of money from domestic sources and from equation (10) may be written as:

L_ct = N_t-1  + C_rt - aL_ct-1                                                    (14) 

The following equation combines the two sources of money given by equations (13) and (14).  We can retain a single variable (N_t-1) for the domestic production for the domestic economy in period t-1:

L_t = N_t-1 + C_rt - aL_ct-1 + X_t-1                                           (15)

This equation for the money income constitutes the money that is be spent on domestic products and imports as shown in equation (4)  [L_t = N_t  + M_t].  As in equation (6) [L_t = N_{t  +} m(N_t-1   + X_t-1)] we can apply the marginal propensity to import "m" to the money created from all sources.  The loan repayments factor relates only to domestic debt, the domestic source of money. 

This model is presented in Figure 3.  Exports are assumed to be $5 B and the marginal propensity to import "m" is 25% of the money spent.  Bank credit "C_rt" is assumed to be $5 B also, and the rate of loan repayments "a" is 25% of domestic loans outstanding with the banks. 

     

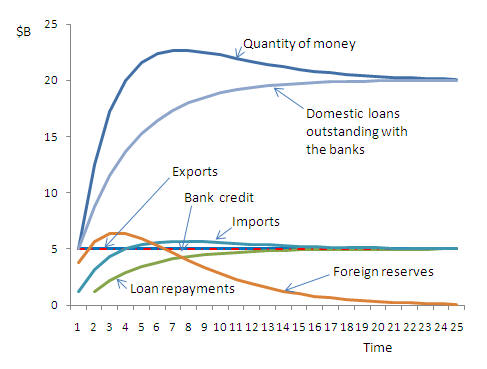
Figure 3: Interaction of Foreign and Domestic sources of money

Although the two sources of money are combined in this model, the equilibrium quantity of money remains at $20 B.  The current account balance can be traced by following the difference between imports and exports and the slope of the foreign reserves curve.  Initially, the current account is in surplus with exports exceeding imports.  After period 4 there is a current account deficit with imports exceeding exports. From period 8, imports start to decline and approach the level of exports.  In equilibrium, imports equal exports, the current account is balanced and foreign reserves are zero.

The zero balance in the level of foreign reserves revealed in this special example provides a key to understanding the cause of the current account deficit.  We saw in Figure 1 that the equilibrium level of money from foreign sources was $20 B and this created foreign reserves of an equivalent amount.  That money was created because the economy saved.  It sold more than it purchased because it exported more than it imported. 

The money created by bank credit does not come from saving: it comes from lending.  As was explained above, the money people earn from selling goods and services enables them to buy products of equivalent value to that which they had produced (and sold).  If additional money is created, it enables the economy to buy more than it has produced.  In our analogy of the airline, bank credit to issuing additional tickets. In "The quantity of money" paper, money created by the growth of bank lending is defined as "unendowed" money.   

Balancing the current account requires the balancing of the foreign and domestic sources of money.  That does not mean that we need equal quantities of money from the two sources.  In the Figure 3 example, the net quantity of money created from foreign reserves was zero and there was $20 B created from bank credit.  The element that must be balanced is the equilibrium level of money from foreign reserves relative to the equilibrium level of money from domestic sources.  From equations (7) [L^* = X/m ] and (11) [L^* = C_r/a], the equilibrium current account balance can be put as:

CAB = X/m - C_r/a                                                         (16)

Where "CAB" means the current account balance.

In the the example associate with Figure 3, the equilibrium quantity of money from foreign trade was $20 B and the equilibrium quantity of money from domestic sources was also $20 B.  As a result the current account was balanced in equilibrium with foreign reserves of zero.

Current account deficit and money

If we assume that the equilibrium quantity of money from domestic credit is greater than the equilibrium quantity of money from foreign sources the outcome will be a net current account deficit in the long term.  Figure 4 assumes that the rate of loan repayment has reduced from 25% per period to 12.5%.  This is equivalent to increasing the average term of bank loans from 4 periods to 8 periods.    

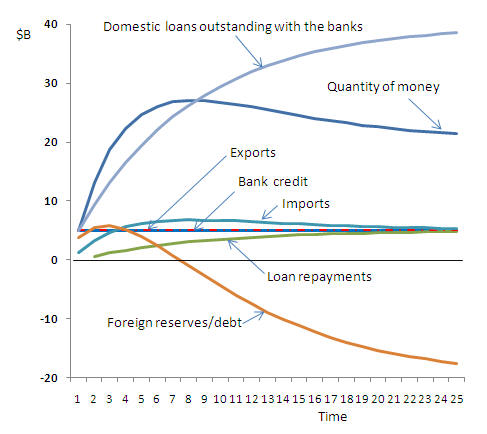
Figure 4: Current account deficit

In the Figure 4 example, the economy has a current account surplus for the first 3 periods after which the current account balance turns to a deficit.  When the economy reaches equilibrium, the current account is balanced, but there is an accumulated foreign debt of $20 B. 

Applying the formula for the current account balance, the equilibrium quantity of money from foreign sources would have been $20 B (5/0.25).  The equilibrium quantity of money from domestic sources would have been $40 B (5/0.125).  While the current account has stabilized, at the end of the time series the current account balance is a deficit of $20 B. 

Some of the features of this economy is that the growth of bank credit is greater than the income of the economy.  Also, it is evident that the amount by which the growth of bank loans exceeds the quantity of money is equal to the level of foreign debt.

Current account surplus and money         

In the following example, we will assume that the equilibrium quantity of money from bank credit is less than the equilibrium quantity of money from foreign sources.  To do so, we will assume that the rate of loan repayment is 0.4, equivalent to a term of two and a half periods for the average loan.  All the other variables remain as they were.  Applying equation (16) [CAB = X/m - C_r/a] we can determine that the equilibrium quantity of money from domestic sources would be $12.5 B while the equilibrium quantity of money from foreign reserves remains at $20 B.  Therefore, the long term current account surplus would be $7.5 B.  This is evident in Figure 5 below. 

 

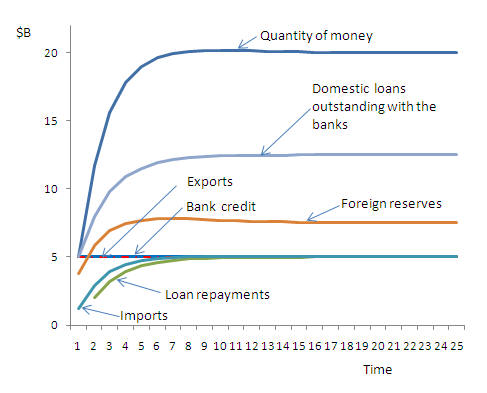
Figure 5: Current account surplus

The example presented in Figure 5 differs from Figure 4 in that the growth of domestic loans outstanding with the banks is now less than the growth in the total quantity of money.  Foreign reserves have contributed to the quantity of money.   

The rate of repayment of bank loans is particularly significant to the equilibrium level of money from bank credit.  The longer the term of the loans, the more likely it is that the equilibrium quantity of money from bank credit is greater than the equilibrium quantity of money from foreign reserves.

This relationship suggests that a country with a current account deficit should aim to reduce the term of bank loans.  Commercial or trading bank credit should not be used for long term loans such as home mortgages.  Other forms of finance should be used for such loans (e.g., savings bank loans, superannuation funds, etc).

Current account balance and capital flows   

International capital flows also have an effect on the quantity of money in an economy with fixed exchange rates.  We can include capital inflow to equation (15) such that:

L_t = N_t-1 + C_rt - aL_ct-1 + X_t-1 + K_t-1                                     (17)

Where:

K_t-1 is the net foreign capital inflow in the previous period.

Foreign capital inflow adds the quantity of money in much the same way as increased exports. The equilibrium quantity of money can be put as:

 L^* =  (X + K)/m                                                            (18)

However, capital flows are not income.  They represent an increase in foreign debt or an increase in foreign equity in the economy.  If we were to consider only the balance of payments, that is, the level of foreign reserves, they could be but put as:

 R_t = R_t-1  + X_t-1  + K_t-1 - M_t-1                                        (19)                          

Drawing on equation (16) [CAB = X/m - C_r/a], the equilibrium level of foreign reserves can be put as:

 R^* =  (X + K)/m   - C_r/a                                               (20)

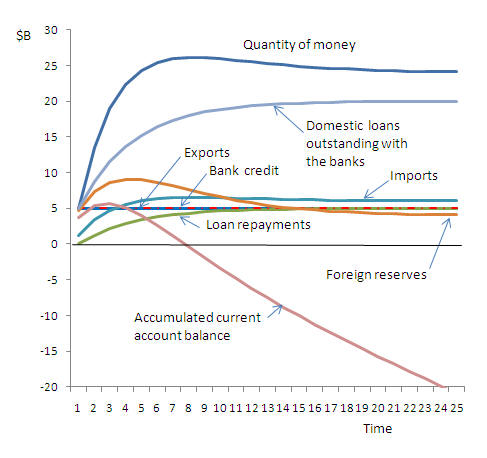
Figure 6: Net capital inflow and the current account balance

When compared to the example in Figure 3, the capital inflow has increased the equilibrium quantity of money by $4 B to $24 B.  Also, the equilibrium level of foreign reserves has increased from zero to $4 B, consistent with equation (20).  However, the economy experiences a perpetual current account deficit. So the current account balance formula (16) [CAB = X/m - C_r/a] can be modified by subtracting the accumulated capital inflow such that:

CAB = X/m - C_r/a - sK                                               (21)

Where:

sK is the sum of foreign capital inflows over the relevant period (foreign debt and foreign equity).

Essentially, this equation is saying that the current account balance is equal to national savings (X/m) less the growth in bank credit (C_r/a), less international borrowing (sK).

The same approach can be applied to assess the impact on an economy of net capital outflow.  In Figure 7 the value of capital (K) which was +1 in Figure 6 is changed to -1, representing a net capital outflow.  This lowers the equilibrium quantity of money from $20 B to $16 B, consistent with equation (18).

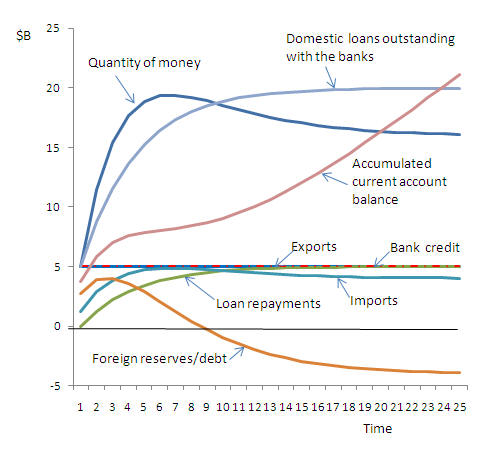
Figure 7: Net capital outflow and the current account balance

Consistent with equation (20) [R^* =  (X + K)/m   - C_r/a] official foreign reserves have fallen below zero to stabilize at a foreign debt of $4 B.  Following the decline in the quantity of money available to spend, the equilibrium level of imports has fallen from $5 B to $4 B.  The economy experiences current account surpluses which stabilize at $1 B per period.  The accumulated current account surplus continues to rise while the capital outflow continues. 

The current account balance with floating exchange rates

The analysis above reveals the significance of financial deregulation on countries with fixed exchange rates.  That is, increasing total bank lending lowers any current account surplus, raises any current account deficits and could turn an economy with current account surpluses into one with a current account deficits.

It also suggests that devaluing a currency would help to reduce a current account deficit if it reduces the value of "m", the marginal propensity to import.  Countries have moved to the floating exchange rate system expecting that the exchange rate would move to balance the current account.

However, the floating the exchange rate system has not eliminated the current account deficit.  Rather, the relationship between the current account and bank credit has become even more explicit as can be observed in the relationship between bank credit and the accumulated current account deficit for Australia, New Zealand, India, the Philippines and the USA.  

The following analysis initially considers some of the popular myths about the floating exchange rate.  It then proposes an explanation on the relationship between the current account and the growth of bank credit that is consistent with the statistics.  Finally it presents a variation of the floating exchange rate system that provides opportunities to balance the current account while maintaining market determined exchange rates.

Floating exchange rate achieves external balance hypothesis

Some proponents of the floating exchange rate system believe that the exchange rate should adjust to bring about a balance between the foreign receipts and payments.  To assess that hypothesis, let us assume that the exchange rate does adjust to balance imports and exports.  To start with we will assume that there are no capital inflows. We will assume the same economy presented in Figure 3 above but with floating exchange rates.

In that case, exports would be given by:

X_t = X/e_t                                                                    (22)

Where: 

e_t  is the exchange rate at time "t"; and

X  is the value of exports in terms of foreign currency, which is assumed to be constant.

This means that if the exchange rate appreciates, the value of exports will fall in terms of domestic currency.  If the currency depreciates, the value of exports will rise in terms of domestic currency.

We will assume that imports are given by:

M_t = e_tmL_t                                                                 (23)

That is, the higher the exchange rate, the greater the imports. 

Without capital flows, under the floating exchange rate system, imports would equal exports.  That is:

            M_t = X_t                                                                 (24)

Substituting equations (22) and (23) into equation (24) means that:

            e_tmL_t = X/e_t                                                         (25)

Which can be rewritten:

            e_t² = X/mL_t

            e_t   = √(X/mL_t)                                                      (26)

Substituting equations (22) into equation (15) [L_t = N_t-1 + C_rt - aL_ct-1 + X_t-1] provides the equation for the quantity of money available to be spent in the economy. 

L_t = N_t-1 + C_rt - aL_ct-1 + X/e_t-1                                      (27)

This money is spent on domestic products and imports.  As in earlier models, imports increase with the quantity of money.  But this time the exchange rate depreciates over time to adjust exports up and imports down so that they remain equal. The outcome is modelled and presented in Figure 8 using the exchange rate presented in equation (26).   

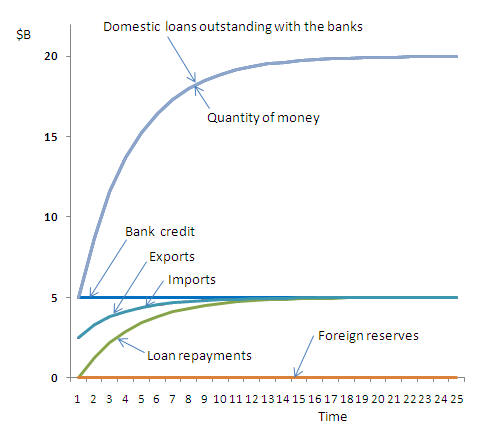
Figure 8: Credit growth with floating exchange rates

In this model, there is always external balance so there is no injection of money from exports or leakage of currency to imports.  As in Figure 3, the quantity of money grows to $20 B, following the introduction of bank credit of $5 B per period and repayments equal to 25% of loans outstanding.  All money is created from bank credit so that the quantity of money is equal to the domestic loans outstanding with the banks.

This outcome is similar to the equilibrium outcome in Figure 3. Yet while it is theoretically possible, and possible to model, there is no sustained period of time in the data for Australia, New Zealand, the USA, India and the Philippines where there is evidence of such an outcome.  Therefore, the exchange rate is not having this effect that is being modelled.  The floating exchange rate system is not bringing about external (international) balance in these countries.  

Floating exchange rates and the capital inflow hypothesis

The other common explanation for the current account deficit is that it is caused by foreign capital inflows.      

Let us continue with the floating exchange rate model used to derive Figure (8) but assume that foreign investors wish to invest $1 B of foreign currency, as was assumed in Figure (6) with fixed exchange rates. 

In that case, foreign currency receipts would be made up of exports and foreign capital given by:

X_t+ K_t = (X_t+K_t)/e_t                                                       (28)

Where  "K"  is the value of foreign capital inflow in terms of foreign currency, which is assumed to be constant. 

Under the floating exchange rate system imports must equal exports plus foreign capital inflow.  That is:

M_t = X_t + K_t                                                               (29)

Substituting equations (22) and (28) into equation (29) defines the equilibrium situation of imports equalling exports and foreign capital with the exchange rate adjusting to bring about balance.    

e_tmL_t = (X_t+K_t)/e_t                                                        (30)

Solving this equation for "e" determines the exchange rate to achieve this outcome to be:

e_t² = (X+K)/mL_t

e_t   = √((X+K)/mL_t)                                                      (31)

Substituting equation (28) into equation (17)[L_t = N_t-1 + C_rt - aL_ct-1 + X_t-1 + K_t-1] provides the equation for the money available to be spent in the economy.

L_t = N_t-1 + C_rt - aL_ct-1 + X/e_t-1   + K/e_t-1                             (32)

Again, the money available is spent on domestic products and imports.  Using the exchange rate presented in equation (31), the monetary income shown in equation (32) and assuming a capital inflow of $1 B, the modelled outcome is presented in Figure 9.

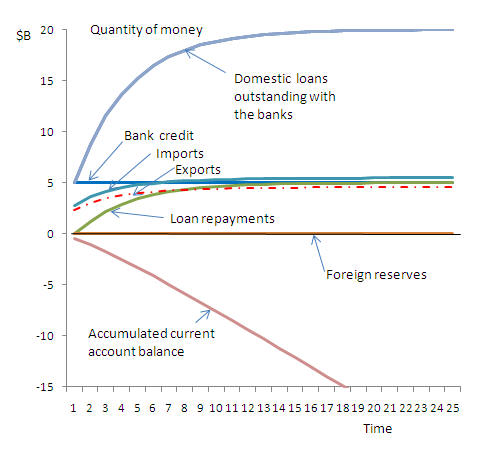
Figure 9: Capital inflow generating current account deficits  

The current account deficit is the amount by which imports exceed exports and it is accumulated each period as the "Accumulated current account deficit" line. This is equal also to the accumulated capital inflow which is an indicator of the level of foreign debt and equity accumulated.  It is clearly evident that Figure 9 and the associated theory and model does not reflect nor explain the relationship between the current account deficit and bank credit that is evident in the data for Australia, New Zealand, the USA, and the Philippines.

In all four countries, the current account deficit is generally equal to the growth of bank credit.  Although the exchange rate has varied in each of these countries over the period shown, those variations have not affected the basic relationship. Therefore, the capital inflow hypothesis is  not a valid explanation of behaviour of the economy under floating exchange rates.

The demand and supply constraint hypothesis

The quantity of money available to be spent can be defined in the same way under the floating exchange rate system as under the fixed exchange rate system.  That is, equation (17) still holds under the floating exchange rate system:

      L_t = N_t-1 + C_rt - aL_ct-1 + X_t-1 + K_t-1                               (17)

The major change under the floating exchange rate system is the requirement that international payments and receipts must be equal. That is:

M_t = X_t + K_t                                                               (29)

It is evident from the statistics for countries with floating exchange rates such as Australia, New Zealand, the USA and the Philippines that the current account deficit is equal to the growth of money from bank credit (bank loans less loan repayments).  That is:

M_t - X_t = dC_rt                                                            (33)

Where dC_rt is the growth in bank credit in a period and is equal to bank credit in a period less loan repayments (of principal) in that period which is given by:

dC_rt = C_rt - aL_ct-1                                                       (34)

The reason why the current account deficit should equal the growth in bank credit is not so clearly evident. However, in equation (3) we defined income in a period "t" as:

Y_t = N_t  + X_t                                                              (3)  

The only way an economy with a floating exchange rate (without additional money from foreign sources) can spend more than it earns is by creating additional money from bank credit.  Therefore, we can say that total money available to be spent is equal to income plus the growth in bank credit:

L_t = Y_t + dC_rt                                                            (35)

If we substitute equation (3) into equation (35) we can say that the money we have to spend is equal to exports plus bank credit:

L_t = N_t  + X_t + dC_rt                                                     (36)

We assumed earlier in equation (4) that we spend our money on domestic products and imports:

L_t = N_t  + M_t                                                            (4)      

Therefore, substituting equation (36) into equation (4) we can say that:

 N_t  + X_t + dC_rt = N_t + M_t                                            (37)

This is the demand and supply constraint hypothesis.  The left hand side of the equation represents the demand constraint, the money available to be spent.  The right hand side of the equation represents the supply constraint; what can be bought.  It can be simplified to:

X_t + dC_{rt =} M_t                                                            (38)

In equation (38) the left hand side of the equation represents the money that is available to be spent on the foreign exchange market.  The right hand side represents what it can be spent on.  It can be rewritten as equation (33);

M_t - X_t = dC_rt                                                             (33)

This is the standard Keynesian explanation for the current account deficit in which the growth in credit is the amount by which investment exceeds saving and government expenditure exceeds taxes.  The only way that investment can exceed saving and government expenditure exceed taxes is to add to the domestic money supply.  That is, to raise bank credit.

Substitution equations (22) [X_t = X/e_t] and (23) [M_t = e_tmL_t] into equation (33) means that:

            e_tmL_t - X/e_t = dC_rt                                                 (39)

which may be rewritten as:

            e_tmL_t - dC_rt - X/e_t = 0                            

            e_t²mL_t - e_tdC_rt - X = 0                                           (40)

Equation (40) is in the quadratic form "ax² + bx + c =0" which can be solved with the standard formula for solving a quadratic equation.  Therefore, the exchange rate that solves equation (40) is:

e_t = (dC_rt +/-  √(dC_rt² + 4mLX) )/(2mL_t)                         (41)

                        

Equation (17)[L_t = N_t-1 + C_rt - aL_ct-1 + X_t-1  + K_t-1], can be restated by substituting equation (34) for credit growth, equation (22) [X_t = X/e_t] for exports and equation (23) [M_t = e_tmL_t] for imports such that:

L_t = N_t-1 + dC_rt + X/e_t-1  + K_t-1                                         (42)

By using equation (41) for the exchange rate, we can ensures that the external balance in terms of foreign currency is maintained.  That is:

M_t = X_t + K_t                                                               (29)

It also ensures that internal balance is maintained in terms of domestic currency.  That is:

M_t = X_t + dC_rt                                                            (38)

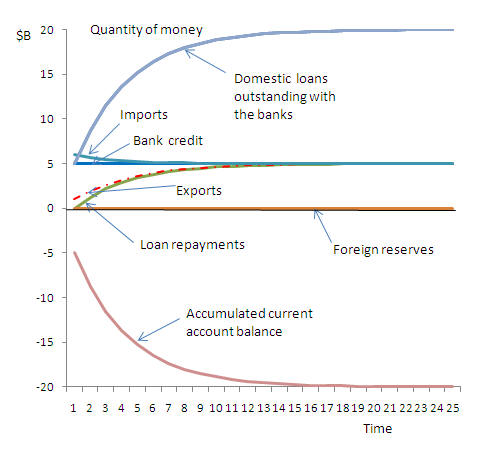
Figure 10: Money supply and current account balance with floating exchange rate system     

The relationship present in Figure 10 is a product of the floating exchange rate system.

To compare the quantity of money with the current account balance, Figure 11 plots the same relationship as in Figure 10 but with the current account deficit (the inverted current account balance) and shows the foreign capital inflow.

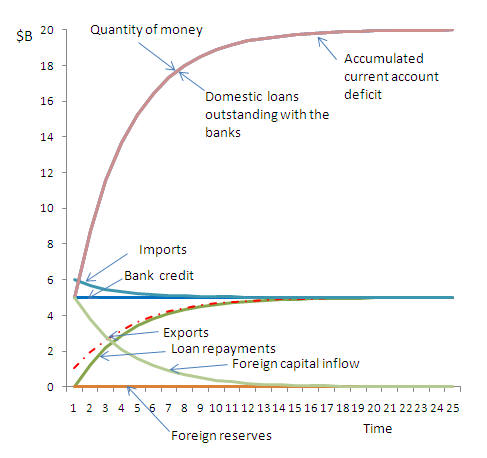
Figure 11: Money supply and current account deficit with floating exchange rate system     

Figure 11 shows how the difference between imports and exports is met by foreign capital inflow.  Also, foreign capital inflow is equal to the growth of bank credit.  However, foreign capital does not normally drive the outcome. It is bank credit that drives the flows in the studied countries. 

The demand and supply constraint hypothesis with net private capital outflow

While the supply constraint hypothesis may apply to countries such as the USA and Australia with net capital inflow, the question arises as to whether it explains the outcomes of countries such as Japan that have a current account surplus. 

To asses the outcome, we will assume that the economy spends not only on domestic products and imports but makes a fixed foreign investment (private capital outflow).  Therefore, we will modify equation (4) such that spending is given by:

L_t = N_t  + M_t + K_ot                                                       (43)      

Where: 

K_ot is a fixed amount of capital outflow that is invested in each period "t".

In a similar approach to that used to create equation (37), we substitute equation (36)[L_t = N_t  + X_t + dC_rt] into equation (43) to determine that:

 N_t  + X_t + dC_rt = N_t + M_t + K_ot                                    (44)

As in equation (37), the left hand side of the equation represents the demand constraint, the money available to be spent.  The right hand side of the equation represents the supply constraint; what can be bought, including foreign investments.  Equation (44) can be simplified to:

X_t + dC_{rt =} M_{t +} K_ot                                                   (45)

As in equation (38) [X_t + dC_{rt =} M_t] the left hand side of the equation represents the money that is available to be spent on the foreign exchange market.  The right hand side represents what it can be spent on.  It can be rewritten as:

M_t - X_t = dC_rt - K_ot                                                     (46)

In this equation, the capital outflow represents national savings.  Therefore, the current account deficit is to the growth of bank credit less national savings.

Substituting equations (22) [X_t = X/e_t] and (23) [M_t = e_tmL_t] into equation (46) means that:

            e_tmL_t - X/e_t = dC_rt  - K_ot                                        (47)

which may be rewritten as:

            e_tmL_t - (dC_rt- K_ot) - X/e_t = 0                            

            e_t²mL_t - e_t(dC_rt - K_ot) - X = 0                                  (48)

Equation (48) can be solved with the standard formula for solving a quadratic equation.  Therefore, the exchange rate that clears the foreign exchange market is given by:

e_t = ((dC_rt- K_ot) +/-  √((dC_rt- K_ot)² + 4mLX ))/(2mL_t)        (49)

The money available to be spent continues to be given by equation (42) as the private capital outflow is not directly available to the domestic economy.  The private capital outflow reduces income from domestic sources (N_t-1) and raises foreign investment (K_t-1).  This can be seen in the spreadsheet (Figure 12, Columns P and J).

      L_t = N_t-1 + dC_rt + X/e_t-1  + K_t-1                                            (42)

Figure 12 plots the outcome of this model using the exchange rate equation (49).  This model is similar to that used for Figures 10 and 11 except that it assumes investment overseas of $1.2 B in each period.    

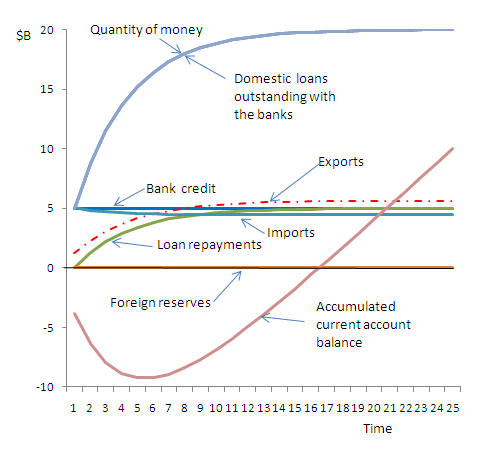
Figure 12: Private capital outflow and current account balance with floating exchange rate system

Initially, the growth in net bank credit is greater than the capital outflow.  This causes current account deficits.  However, when the loan repayments increase so that the investment overseas exceeds the growth of bank credit, the current account balance is reversed and the economy experiences current account surpluses.  This is the experience of countries such as Japan whose net capital outflow is greater than the net growth in bank credit.

The demand and supply constraint hypothesis with net official capital outflow

The Central Bank of the Philippines has intervened in the floating exchange rate system to stabilize the exchange rate of the Peso.  In the process its foreign reserves have been growing and the Philippines have been experiencing current account surpluses. 

The following model extends the Figure 12 model to explain what is happening in the case of economies such as the Philippines that significantly increase their foreign reserves.

In an economy with private capital outflow, the investment reduces the expenditure on other products.  However, when the banking system initiates capital outflow, it does not reduce expenditure on other products.  That is, instead of equation (43) we can return to equation (4).  That is, money can be spent on domestic products or imports:

L_t = N_t  + M_t                                                              (4)    

This is the only difference between private capital outflow and official capital outflow that raised foreign reserves.  In the case of private capital outflow, from equation (43)[L_t = N_t  + M_t + K_ot] we can say that expenditure on domestic products can be put as:

N_t-1 = L_{t-1  -} M_t-1 - K_ot-1                                              (50)   

In the case of official foreign investment, from equation (4) we can say that:

N_t-1 = L_{t-1  -} M_t-1                                                        (51)

This change alters the value of "N_t-1" in equation (42), that is, in:

       L_t = N_t-1 + dC_rt + X/e_t-1  + K_t-1                                         (42)

The foreign exchange market does not distinguish between private and official capital flows. Therefore, it is possible to retain the equation used to define the exchange rate in the private capital outflow example, equation (49)                         [e_t = ((dC_rt- K_ot) +/- √((dC_rt- K_ot)² + 4mLX ))/(2mL_t)], except that we will replace private capital outflow with official capital flows that raise the foreign reserves of the banking system.  That is: 

e_t = ((dC_rt- dR_t) +/-  √((dC_rt- dR_t)² + 4mLX ))/(2mL_t)        (52)

                                                       

Where:

dR_t is the growth in the foreign reserves of the banking system in time "t".

Figure 13 plots the effect of this change.  In this example, all the variables have the same values except that instead of private capital outflow of $1.2 B per period, the foreign reserves are increased $1.2 B in each period.

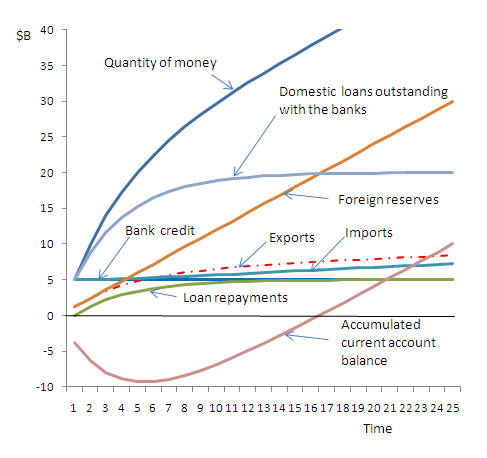
Figure 13: Official capital outflow and current account balance with floating exchange rate system

As in the example with private capital outflow, the country experiences a current account deficit while the growth of bank credit is greater than the growth in foreign reserves.  When the growth in foreign reserves is greater than the growth in bank credit, the economy experiences a current account surplus. 

The major difference also evident is that the growth in foreign reserves adds to the quantity of money and stimulates the economy in a way that raises foreign reserves and brings about a current account surplus.

Given the massive debts accumulated by governments to stimulate their economies during the global financial crisis, the use of official capital outflow to stimulate the economy would avoid the debt burden and actually reduce net foreign debt. 

Comment 

This paper has shown that it is possible to find a unifying theory and a formula explaining the current account balance for countries with both fixed and floating exchange rates. The Demand and Supply Constraint Hypothesis is able to explain both current account deficits and current account surpluses under the floating exchange rate system.  Also, it is able to explain the effect of different types of capital flows.  These models are reflecting the behaviour of real economies and provide a reasonable foundation to evaluate alternative policies for managing economies.

To come back to the initial analogy, the monetary system economy is more complex than a simple airline ticketing system.  Yet the basic principle holds true that if a country issues more money than its own ability to honour that money, it will accumulate current account deficits that can lead to large foreign debts.

The models used have been simple, in most cases without any growth in exports or credit.  They are used as a teaching tool, not as a model of any particular economy.  As mentioned earlier, the Excel workbook modelling these outcomes and used to generate the above graphs is available.

The Optimum Exchange Rate System

It is possible to restructure the financial system so that financial institutions, acting in their own interest, will ensure balance of payments stability.  The optimum exchange rate system and the guided exchange rate and liquidity system are exchange rate systems that take into account the significance of monetary growth for the external balance.  Both systems manage the growth of bank credit so that the money from bank credit does not cause balance of payments difficulties.

In the following example, I consider the optimum exchange rate system.  The system has two parts: one that manages the growth of bank credit so as to ensure balance of payments stability; and the second part manages the exchange rate to achieve full employment. `    

To explain the first part, we will return to the model used to present Figure 3.  This model has fixed exchange rates and money is created from both bank credit and foreign reserves.  In this model, the money available to be spent is given by equation (15).

L_t = N_t-1 + C_rt - aL_ct-1 + X_t-1                                           (15)

In the earlier model, the growth of bank credit was assumed to be fixed.  In this case we are going to assume that the growth in bank credit is given by equation (53).

        C_rt = (bR_t-1 - L_ct-1)/b² + aL_ct-1                                          (53)

Where:

b is the maximum bank credit to foreign reserve ratio;

       R_t-1 is the level of foreign reserves at time “t-1”; and

       L_ct is the level of domestic loans outstanding with the banks.

This model is presented in Figure 14.  Exports are assumed to be $5 B and the marginal propensity to import "m" is 25% of the money spent.  The rate of loan repayments "a" is 25% of domestic loans outstanding with the banks.  In this example, the maximum bank credit to foreign reserves ratio is 10.  

 

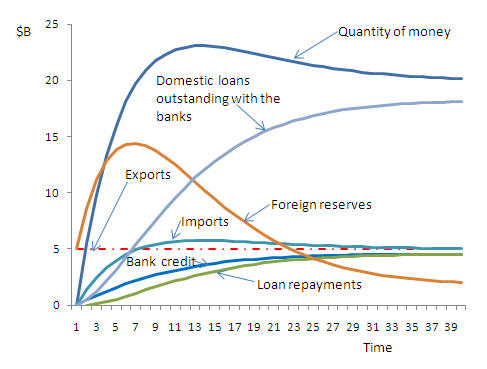
Figure 14: Economy with managed growth of bank credit

In this model, it is not necessary to assume that there are private foreign capital movements.  Hence official foreign reserves also represent the accumulated current account balance.  Initially, both exports and bank credit grow to stimulate the economy.  Then, when imports exceed exports, foreign reserves decline.  Bank credit continues to grow and demand in the economy stabilizes at $20 B.  At equilibrium, foreign reserves are $1.82 B and domestic loans outstanding with the banks rise to $18.2B; ten times the level of foreign reserves. While foreign reserves are one tenth of bank debt, they represent 36 per cent of the level of imports in a period.  

In this example, it has been necessary to increase the time line to 40 periods to show the stable equilibrium outcome.     

The second part of the optimum exchange rate system manages the exchange rate to achieve economic objectives such as full employment.  In this example we will assume that the economic objective is full employment and incentives are built into the monetary system to achieve that objective.

As in the model for Figure 14, we will assume that the money available to be spent is given by equation (15) [L_t = N_t-1 + C_rt - aL_ct-1 + X_t-1]. Substituting equation (53) [C_rt = (bR_t-1 - L_ct-1)/b² + aL_ct-1] into equation (15) reduces it to:

        L_t = N_t-1 + (bR_t-1 - L_ct-1)/b² + X_t-1                                          (54)

 

Also, we will assume that equation (4) [L_t = N_t  + M_t] applies and the money is spent either on domestic products or imports. Substituting equation (4) into equation (54) means that we can say that: 

        N_t + M_t  = N_t-1 + (bR_t-1 - L_ct-1)/b² + X_t-1                                 (55)

In equilibrium, we can say that sales and purchase of domestic products are stable.  That is:

        N_t = N_t-1                                                                              (56)

Also, there would be no growth in bank credit.  That is:

       (bR_t-1 - L_ct-1)/b^{2 =} 0                                                               (57)

Therefore, substituting equations (56) and (57) in equation (55) means we can conclude that in equilibrium:

        M_t  = X_t-1                                                                             (58)

Substitution equations (22) [X_t = X/e_t] and (23) [M_t = e_tmL_t] into equation (58) means that at equilibrium, we can say that:

        e_tmL_t = X/e_t                                                                          (59)

Therefore, the equilibrium exchange rate is given by:

        e_t²   = X/mL_t   

        e_t   = √(X/mL_t)                                                                     (60)       

If the quantity of money required to achieve full employment is represented by L_ft, then we can say that the exchange rate to achieve full employment would be given by:

         e_ft   = √(X/mL_ft)                                                                     (61) 

In Figure 15, we assume that the full employment quantity of money is $30 B.  We will assume that the marginal propensity to import (when the exchange rate is equal to 1), "m"  remains at  0.25. Export income in terms of foreign currency "X" remains at $5 B.  Therefore, the equilibrium exchange rate is equal to √(5/0.25x30) = 0.82.  To avoid excessive inflation, the exchange rate is assumed to move slowly (over ten periods) from 1 to the equilibrium rate, provided that the quantity of money is less than the equilibrium quantity of money.

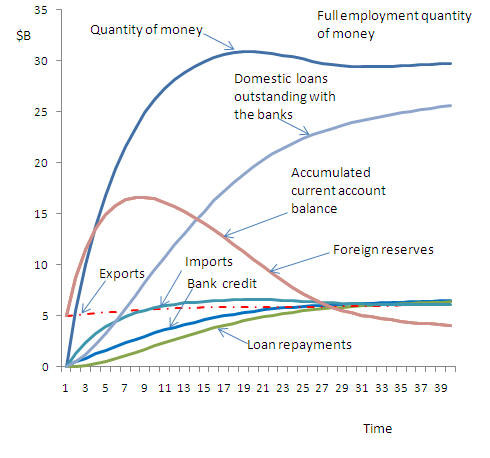
Figure 15: Economy with the optimum exchange rate system

If economies are to grow in a sustainable manner with domestic and international stability, full employment and low inflation, they will need to adopt a monetary system that manages the growth of bank credit and manages the exchange rate, such as the optimum exchange rate system.

In a real situation with the optimum exchange rate system, additional money from export growth may be supplemented with foreign capital.  The inflow of foreign capital may lead to a current account deficit.  When full employment is attained, there would no longer be the same demand for investment and interest rates will fall.  With lower interest rates, foreign capital inflows would decline enabling the attainment of a current account balance.

There may be some economists who continue to believe that the rules relating to bank credit should not be modified to ensure balance of payments stability and current account balance.  They prefer a more liberal approach.

Yet if an airline adopted a "liberal" approach to the issue of airline tickets, allowing agents to issue tickets regardless of the availability of seats, these same economists would condemn the airline for poor management of their ticketing systems. 

Many "responsible" governments persist in allowing their banks to create credit regardless of the disastrous consequences that this is having for their economies.  It is not that these governments are irresponsible, it is that they are not aware of the consequences of their liberal banking laws. 

This paper has shown that persistent current account deficits indicate systemic problems in a country's monetary system.  These problems will not rectify themselves.  Policies and rules were put in place that created these outcomes.  Those policies and rules must be modified if the outcomes are to change.

   

See also: A New European Monetary Union

Money and Inflation, Money and Unsustainable Debt

Growth of Debt and Loss of Income in America

Impact of the floating exchange rate system on employment and growth

Impact of the floating exchange rate system on debt

Saving the Euro

and Free Trade.